Bài Viết

Phép chia có dư là một trong những khái niệm toán học cơ bản nhưng vô cùng quan trọng mà học sinh cần nắm vững ngay từ những năm đầu tiên làm quen với môn toán. Không chỉ đơn thuần là một phép tính số học, phép chia có dư còn là nền tảng để các em hiểu sâu hơn về mối quan hệ giữa các con số và vận dụng vào giải quyết các bài toán thực tế trong cuộc sống hàng ngày.
.jpg)
Hình hộp chữ nhật là một trong những khối hình học ba chiều phổ biến nhất trong cuộc sống hàng ngày. Từ hộp quà, tủ lạnh, phòng học, tòa nhà, đến container vận chuyển hàng hóa, hình hộp chữ nhật xuất hiện khắp nơi xung quanh chúng ta.

Số nguyên âm và dương là một bước tiến quan trọng trong hành trình học toán, mở rộng thế giới số từ những con số đếm đơn giản sang một hệ thống phong phú hơn.
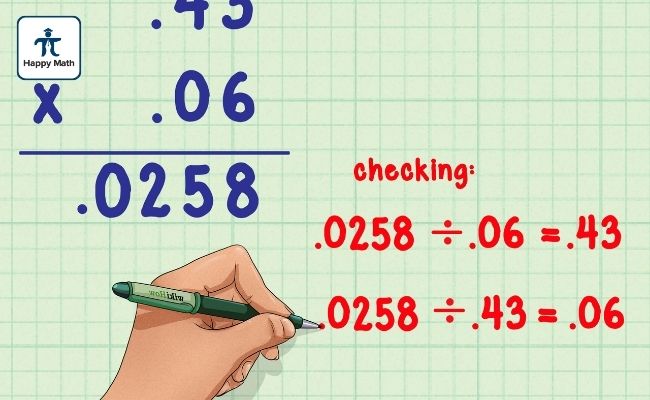
Số thập phân là một phần không thể thiếu trong toán học, xuất hiện từ những bài toán đơn giản trong cuộc sống hàng ngày đến các phép tính phức tạp trong khoa học và kỹ thuật. Việc thành thạo các phép tính với số thập phân không chỉ giúp học sinh nắm vững kiến thức toán học mà còn phát triển tư duy logic và khả năng giải quyết vấn đề.

Phần trăm là một trong những khái niệm toán học được ứng dụng rộng rãi nhất trong cuộc sống hàng ngày. Từ việc tính giá giảm khi mua sắm, hiểu biểu đồ thống kê, đến theo dõi pin điện thoại, phần trăm xuất hiện khắp nơi xung quanh chúng ta.

Hình chữ nhật là một trong những hình học cơ bản và quen thuộc nhất trong cuộc sống hàng ngày. Từ trang giấy trong vở, màn hình điện thoại, cửa sổ nhà, đến sân bóng đá, hình chữ nhật xuất hiện khắp nơi xung quanh chúng ta.
Hình vuông là một trong những hình học cơ bản và đẹp nhất trong toán học, với tính đối xứng hoàn hảo và các tính chất độc đáo. Từ viên gạch lát sàn, cờ vua, màn hình máy tính bảng, đến các tác phẩm nghệ thuật, hình vuông xuất hiện khắp nơi trong cuộc sống và văn hóa của chúng ta.

Phân số là một trong những khái niệm toán học cơ bản và quan trọng nhất mà học sinh bắt đầu tiếp cận từ những năm học đầu tiên. Việc hiểu rõ về phân số đơn giản không chỉ giúp các em nắm vững nền tảng toán học mà còn ứng dụng rộng rãi trong cuộc sống hàng ngày.